ARR, Discounted Payback, NPV, IRR (35.2)
- Thiago Casarin Lucenti
- Feb 7
- 5 min read
Chapter 35 - Investment Appraisal
Learning Objectives: To learn the investment appraisal techniques of ARR, Discounted Payback, NPV, and IRR
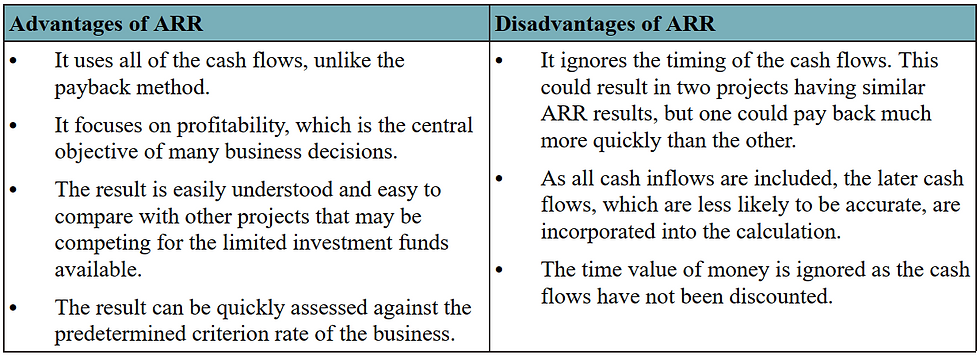
The Account Rate of Return (a.k.a. average rate of return) is another common investment appraisal method. It measures the annual profitability of an investment as a percentage of the initial investment.
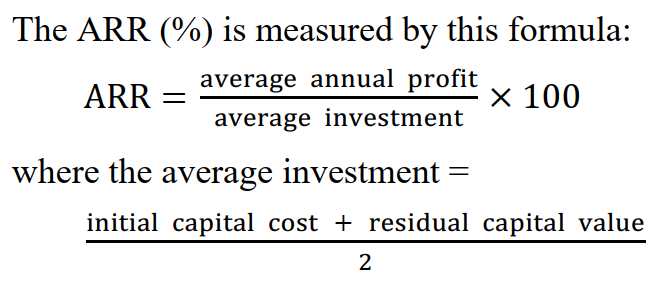
Projects with higher ARR are usually better than the ones with lower ARR;
For simplicity we will assume that the net cashflow = annual profitability of the project;
The net cash inflows are assumed to be = revenues - also for simplicity purposes.
Example:
A company makes a $8,000,000 investment to upgrade their vehicle fleet to more fuel efficient vehicles. Their net cashflow is shown below:
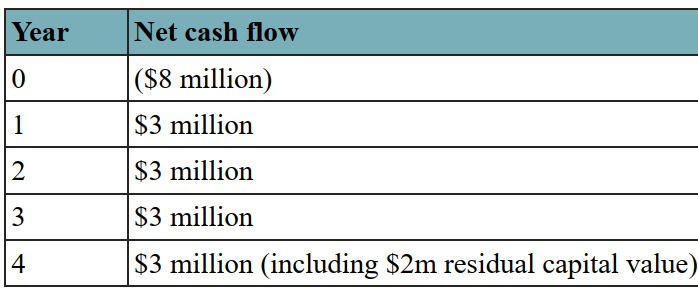
- Year 0 outflow = $8,000,000;
- Years 1 to 3 inflows = $3,000,000 are the annual cost savings with fuel;
- Year 4 inflow = $1,000,000 of fuel savings + $2,000,000 in residual value from selling the vehicles.
To calculate the ARR for this project:
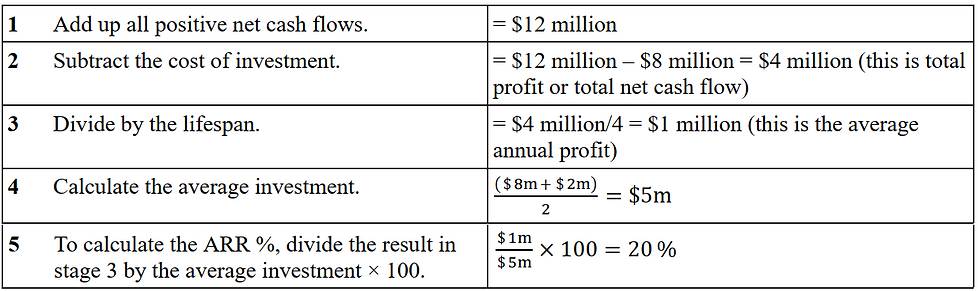
This result indicates that, on average, throughout the lifespan of the project, it will yield 20% of return per year.
What are the uses of such results?
Comparing with alternative projects;
Compare against criterion rate (minimum expected return for a project to receive a green light);
Compare with annual interest rate of debt financing (if ARR is lower then debt financing is not worth it taking for this project);
When used alongside Payback Period it gives a wide view over profits and cashflow timings.
Activity 35.3
What if potential investments show...
Project A is estimated to payback at the end of year 3 at an ARR of 15%
Project B with a payback of 4 years but an ARR of 17%
This is where discounting future cashflows come in for a better assessment. But before going into that, another tricky question:
Ignoring the effects of inflation, would you rather get paid $1000 today or $1000 next year?
Money now is better than money later!
You can rip the benefits of that funding right away instead of waiting for an year;
At interest for an year $1,000 will yield returns;
The future is uncertain: the $1000 offer may be revoked next year.
This is called considering the time value of money in a decision!
Discounting future cashflows does exactly that - it reduces the value of future cashflows so that the it displays the actual value in today's terms.
Here goes another one:
How much less is future cashflow worth compared to today's money?
It all depends on the rate of interest!
Receiving $1,000 today at 10% interest leads to $1,100 in 1 year time;
$1,100 in 1 year time is worth it $1,000 today given a 10% interest;
$1,000 therefore is the present value of $1,100 received in 1 year time.
Discounting calculates the present values of future cash flows so that investment projects can be compared with each other by considering today’s value of their returns.
Let's learn how this is done - the whole process depends on two factors:
The higher the interest rate, the less value future cash has in today’s money;
The longer into the future cash is received, the less value it has today.
Example:
A project has an expected inflow of $3,000 in 3 years;
The current interest rate is 10%.
You will always be given a discount factor table like the one below. You will then need to pick the correct discount factor:

The discount factor for 10% interest in cashflows within 3 years = 0.75 - meaning that in 3 years time $1 is worth the same as $0.75 today.
The present value of this return on investment will be: 0.75 * 3000 = $2,250
- Remember: the discount factor will always be given!
This leads us to the next investment appraisal technique: the Discounted Payback - which is very similar to Payback Period but it considers the time value of money:
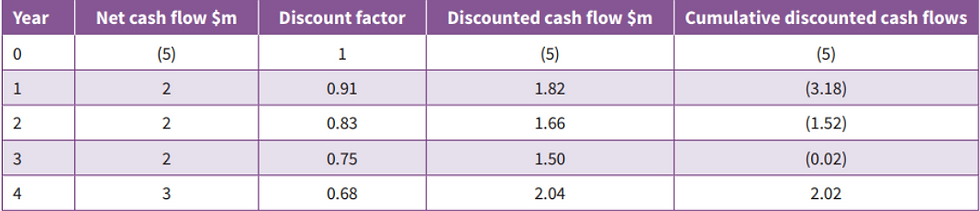
By looking at this table, what would be the time for the "normal" (not discounted) Payback to happen?
After 2 years and 6 months the project would pay back (non-discounted);
What would the discounted payback period be at an interest of 10%?
At the beginning of year 4 - the additional cash needed (0.02) is so small that we could consider the discounted payback period to be 3 full years:
Found by discounting the cashflow and adding them up.
The discounted payback period will always be longer than the simple payback period.
Example 2 @8% - Introduction to NPV:
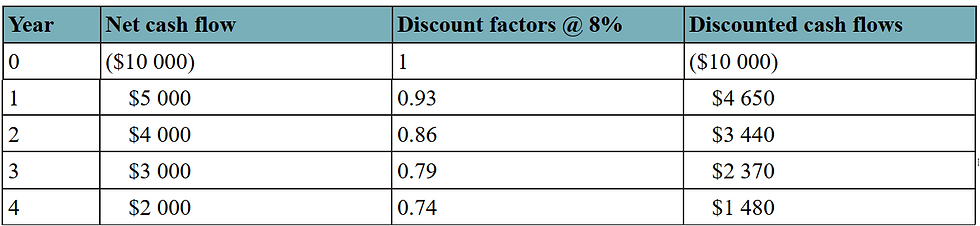
Net Present Value (NPV) refers to today’s value of the estimated cashflows resulting from an investment. It does so by subtracting the capital cost from the total discounted cashflows.
To find your NPV:
Multiply net cash flows by the discount factor (year 0 is not discounted as it is present-value (today) already);
Add the discounted cash flows;
Subtract the capital cost to find NPV.
Total Discounted Cash Flows = $11,490
Capital Cost = $10,000
NPV = $1,940
What does this result mean?
The project earns $1 940 in today’s money values. So if the finance needed can be borrowed at an interest rate of less than 8%, the investment will be profitable.
If interests were to increase the business will have to discount future cash flows at a higher discount rate;
If interests were to increase NPV would decrease;
Even if finance is raised internally businesses will still use the cost of borrowing (interest) for this calculation due to opportunity cost (savings investment);
It can also be compared against a criterion rate.
What if interests were to increase to, let's say, 12%?
Year | Cash Flow | Discount Factors @12% | Discounted Cash Flows (DCF) |
0 | ($10,000) | 1 | ($10,000) |
1 | $5,000 | 0.89 | $4,450 |
2 | $4,000 | 0.79 | $3,160 |
3 | $3,000 | 0.71 | $2,130 |
4 | $2,000 | 0.64 | $1,280 |
The project will still be profitable (although less) - NPV = $1,020.
Points to consider:
NPV is widely used but...
It doesn't show a percentage of return on investment and therefore;
It's used in conjunction with Internal Rate of Return - which is the rate of discount that yields a NPV of zero!
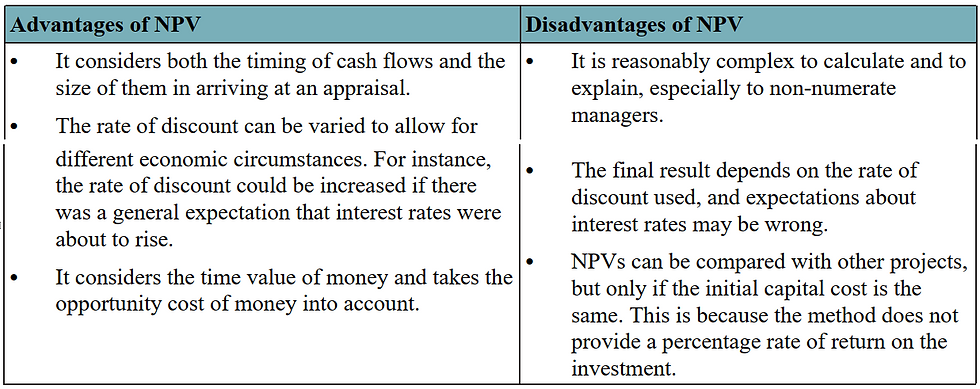
Speaking of IRR...
The Internal Rate of Return (IRR) is the discount rate that makes the net present value (NPV) of a project zero. In other words, it is the expected compound annual rate of return that will be earned on a project or investment.
IRR is usually calculate using a spreadsheet but it can also be estimated through a graph. Let's look into it by considering the discounted cashflows for an investment below:
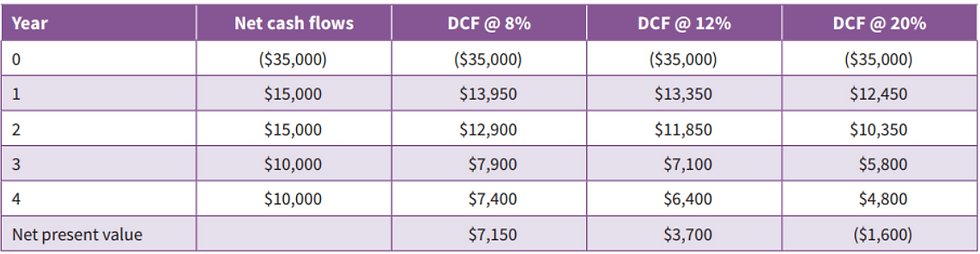
As we know when DCF increases the NPV decreases until it reaches a negative value (passing by zero). You should plot such results on a graph:

Plotting the values you can construct a line;
Where the line crosses the discount rate axis is the IRR.
In other words, it is the expected annual rate of return that will be earned on a project or investment. In the example, an initial investment of $35,000 has a 18% IRR.
Projects costing different amounts can be compared by their rate of return (IRR) and better decisions can be made;
Comparison against interest rate and/or criterion rate becomes easier.
Tedious calculation when done without a computer;
Because it gives an exact result less experienced managers may be led to believe the investment appraisal method is precise and without uncertainties.
To-Do-List
Activity 35.4
Activity 35.5
Chapter 35 - Investment Appraisal



Comments